Making 2-port measurements without S-Params

[The Electrician]

Andrew_K, read this page: https://en.wikipedia.org/wiki/Image_impedance

It is possible to obtain the ABCD parameters by only making one-port impedance measurements.

Consider that you have a two-port.  Make 4 measurements with an impedance analyzer.  Measure the impedances at the input port with the output port first open circuited and then short circuited.  Then measure the impedances at the output port with the input port first open circuited and then short circuited.

Using these formulas, calculate the ABCD parameters:



Since your two-port is reciprocal, only 3 of the 4 measurements are needed to get the 4 ABCD parameters.

[mag_therm]

Hi Virtual,
Thanks for the comments at your #24,  I will dice them up and reply:

Are you suggesting the low impedance tap in order to measure the resonance without loading it with the 50 ohm source of the VNA?
*Yes, that is necessary, as the Q of the inductor would be reduced by the 50 Ohm. In my case the tap was already existing, being the antenna input via a toroidal transformer to a tap on the inductor.

How is the attenuator helpful other than improving the awful source match of the Nano?
* The attenuator on CH0  will use CH1 and LogMag to give the magnitude of 2 versus 1, in my case it showed the peak of the resonance to enable turns adjustment. Also I am not sure, if the nanoVNA can measure LogMag > 0dB. I will check next time it is out.

If the circuit is measured in the Smith Chart mode, measuring S21, impedances will only be accurate from about 20 ohms to 200 ohms for about 5% accuracy.
*I did not try that yet. It probably would not work, as you mention, if Z is high.
*I removed the attenuator and used CH0 to get Smith just on 11  with a sweep over 80 to 20 metre band, re-peaking the tuner at each band.

* To show the method,here are test results on the antenna tuner with the 50 Ohm atten at antenna input. Note that there are taps selected for inductor and variable capacitor is adjusted at each band.
Freq MHz     Smith Z11 Ohm   at resonance      LogMag dB after -20dB atten is added back
3.96        122                                                    +3         
7.07        45                                                             +14
10.05          65                                                             +3
14.04      24                                                            +9

Note that these results will be different when the real antenna is connected, however the tuner can still peak over the above range.

Thanks

[virtualparticles]

Thanks Mag! Appreciate the detailed answer. 

[Andrew_K]

So why must you determine the parameters of each individual block?  Why not just determine the parameters for the whole chain as a single two port?

The reason is threefold

1) I want to examine if I need to change pieces of the signal path.

2) I can cascade the multiple individual blocks and compare against the measurement of combinations as a sanity check.

3) Ease of measurement, the impedance analyzer I was using doesn't do well when the path is too long due to standing waves.

Andrew_K, read this page: https://en.wikipedia.org/wiki/Image_impedance

It is possible to obtain the ABCD parameters by only making one-port impedance measurements.

Consider that you have a two-port.  Make 4 measurements with an impedance analyzer.  Measure the impedances at the input port with the output port first open circuited and then short circuited.  Then measure the impedances at the output port with the input port first open circuited and then short circuited.

Using these formulas, calculate the ABCD parameters:



Since your two-port is reciprocal, only 3 of the 4 measurements are needed to get the 4 ABCD parameters.

This is seriously fascinating.

Did you find those equations in a book somewhere? Or did you derive them youself?
I'd really love to read more, if there is a book or paper.

[The Electrician]

I derived them.

All you need is any three (since your two-ports are reciprocal) of the ordinary two-port parameters.  The driving point (single port) parameters are easy to get with an impedance analyzer; it's the transfer parameters that need another instrument, a gain-phase analyzer.  There are 4 transfer parameters: transfer impedance, transfer admittance, voltage transfer ratio, current transfer ratio.  A gain-phase analyzer can only measure the transfer ratios, not the transfer impedance and admittance.

Suppose you want to get the impedance parameters, z11, z12, z21, z22.   z11 and z22 can be obtained with an impedance analyzer, but z12 and z21 cannot be obtained with a gain-phase analyzer, since they are transfer impedances, not transfer ratios.

But there's another way to get a transfer impedance from a voltage transfer ratio (Av measured with a gain-phase analyzer).

If you have the Z parameters for a two-port, the forward (from input port to output port) voltage transfer ratio is given by Av = z21/z11.

So, if you have z11 (and z22 on the side) and measure forward Av, then z21 = Av*z11.  So now you have z11, z21 and z22; if the two-port is reciprocal, z12=z21, and you have all four Z parameters.  You could then convert the Z parameters to the ABCD parameters.

You need to use the ABCD parameters to be able to calculate the parameters of combined two-ports in cascade.

[Andrew_K]

I derived them.

That's seriously impressive.

I'd love to read a short article about how you did that, if you ever wrote one.

All you need is any three (since your two-ports are reciprocal) of the ordinary two-port parameters.  The driving point (single port) parameters are easy to get with an impedance analyzer; it's the transfer parameters that need another instrument, a gain-phase analyzer.  There are 4 transfer parameters: transfer impedance, transfer admittance, voltage transfer ratio, current transfer ratio.  A gain-phase analyzer can only measure the transfer ratios, not the transfer impedance and admittance.

Suppose you want to get the impedance parameters, z11, z12, z21, z22.   z11 and z22 can be obtained with an impedance analyzer, but z12 and z21 cannot be obtained with a gain-phase analyzer, since they are transfer impedances, not transfer ratios.

But there's another way to get a transfer impedance from a voltage transfer ratio (Av measured with a gain-phase analyzer).

If you have the Z parameters for a two-port, the forward (from input port to output port) voltage transfer ratio is given by Av = z21/z11.

So, if you have z11 (and z22 on the side) and measure forward Av, then z21 = Av*z11.  So now you have z11, z21 and z22; if the two-port is reciprocal, z12=z21, and you have all four Z parameters.  You could then convert the Z parameters to the ABCD parameters.

You need to use the ABCD parameters to be able to calculate the parameters of combined two-ports in cascade.

Understood.

So I have a few options: open/short impedance measurements to form ABCD, impedance analyzer to find h11 and h22 then use a G-P for h12, impedance analyzer to find Z11 and Z22 then find Av to get Z21.

[The Electrician]

Let us know how it goes, periodically.

[Andrew_K]

Using these formulas, calculate the ABCD parameters:


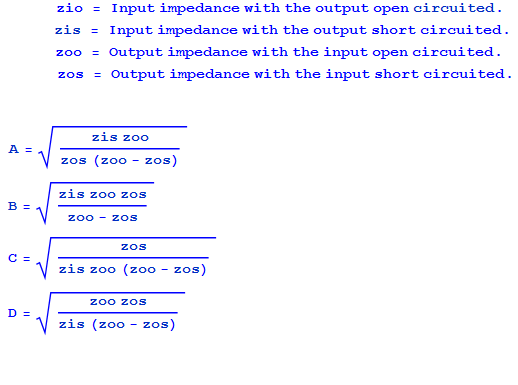
Since your two-port is reciprocal, only 3 of the 4 measurements are needed to get the 4 ABCD parameters.

So, I did try this method.
I think this method should have less measurement error. Changing from Impedance Measurement to Gain-Phase will mean changing fixtures/equipment. Two sets of Calibrations and two sets of systematic error.

One issue I was observing was that open-circuit impedances were garbage at low frequencies. This makes sense, since the impedance approaches infinity at DC, and the impedance measurement is limited.

To remedy this, I decided to add a shunt R at the output. This term preserves the impedance measurement at low frequencies. By measuring DUT+Shunt, then Shunt alone, I should be able to separate out the DUT, in theory...



For clarity, here is my measurement setup:



For the shunt, I did measure Z1O Z1S, Z2O Z2S, and confirmed that the network is reciprocal and symmetrical.




The ABCD for the DUT+Shunt, and Shunt, both look reasonable on their own.

When I attempt to remove the shunt by division, something strange happens. Here is the A parameter of the isolated DUT matrix. There are a few discontinuities in both the A and C parameters.



I think I have isolated how this is happening.

Since the shunt is of the form (The measured shunt is approximately this)
$$ \begin{bmatrix} 1 & 0\\ Y & 1 \end{bmatrix}$$

The equation for the DUT without Shunt is:
$$ \begin{bmatrix} A*1 + B * -Y & A*0 + B*1\\ C*1 + D * -Y & C*0 + D*1 \end{bmatrix}$$

So the $$A-B*Y$$ and $$C-D*Y$$ have a subtraction in them. Complex add/sub seems to give some messy results. I plotted A, B*Y, and the subtraction to better understand.



The discontinuity seems problematic. Still, I continued to see what the final results would look like.

As a sanity check, I wanted to compare the individual measurements with the measurements of the entire network:



I used the ABCD of the shunt, removed it from the ABCD of DUT1, DUT2, and DUT1&2.

Then I calculated DUT1 * DUT2 (cascading) to get the calculated DUT1&2

Here are the plots:



While they roughly have a similar shape, they do not match as I would expect.

There must be measurement error somewhere. The low frequency, low-impedance measurements in the shunt and low-z DUT, seem to have a slight imaginary component where there shouldn't be. This causes significant phase shift at very low Z.

I think my next step will be to try measuring only high-frequency response with the impedance analyzer. Then, use DC measurements to fill a point at, say, 0.1Hz. Then use curve-fitting to generate the transfer function equation I am after.

[The Electrician]

The formulas I gave for the ABCD parameters have an aspect that is not conducive to numerical robustness.  In the denominator of each expression is the difference zoo-zos.  This is the difference between a large quantity and a small quantity.  Not a good thing to have in any formula.

When I've used these formulas, since only 3 measurements are needed to get the four parameters, one can choose which 3 measurements.  I found that not using zio was a good choice, since my input port was a high impedance port, and parasitics tend to seriously contaminate the measurement.

It has occurred to me that it might be better to make the measurements on each port with the two conditions on the other port being not open and short, but with a moderately high impedance load and a short.  Using a moderately high impedance rather than an open would, hopefully, decrease the contamination by parasitics.

I think I was working on deriving the formulas for this case, but I didn't finish.  I'll see if I can find my unfinished work.

In the meantime, the formulas I gave you are theoretically correct, but may not be as numerically robust as one might want. 

[The Electrician]

You have a result of:
[A∗1+B∗−Y A∗0+B∗1]
[C∗1+D∗−Y C∗0+D∗1]

after multiplying the ABCD matrix:

[A B]
[C D]

Times the matrix for a shunt Y, which is:

[1 0]
[Y 1]

How did the result end up with the minus sign in front of the Y?  I don't get that; I get:

[A+BY   B]
[C+DY   D]

[Andrew_K]

The formulas I gave for the ABCD parameters have an aspect that is not conducive to numerical robustness.  In the denominator of each expression is the difference zoo-zos.  This is the difference between a large quantity and a small quantity.  Not a good thing to have in any formula.

When I've used these formulas, since only 3 measurements are needed to get the four parameters, one can choose which 3 measurements.  I found that not using zio was a good choice, since my input port was a high impedance port, and parasitics tend to seriously contaminate the measurement.

It has occurred to me that it might be better to make the measurements on each port with the two conditions on the other port being not open and short, but with a moderately high impedance load and a short.  Using a moderately high impedance rather than an open would, hopefully, decrease the contamination by parasitics.

I think I was working on deriving the formulas for this case, but I didn't finish.  I'll see if I can find my unfinished work.

In the meantime, the formulas I gave you are theoretically correct, but may not be as numerically robust as one might want. 

Welp, that makes a lot of sense! That matches what I was observing. Impedances too high (full open) or too low (full short) were being contaminated by parasitics.

Perhaps the solution to make the 1-port-impedance method work would be using an L pad (resistive) attenuator instead of just a shunt. That way the Open and Short circuit impedances are guarded, rather than just the open-circuit found in a shunt. Then, the attenuator block could be measured and removed by matrix division. I believe that (should) keep the ZOO-ZOS term reasonable?


One other interesting thing I observed: the open-circuit impedance is actually lower than the short-circuit impedance for some frequency range. Initially I thought that shouldn't be possible. Playing around in LT Spice, I found I could replicate the effect with LC Resonators. A small R could detune the resonance and cause the impedance to be higher than if the low R wasn't present at all.

Perhaps that could be another source of instability in my measurements. ZOO and ZOS may cross... At the least, they will approach at high frequency (in my case).


The active probe I have, which I have used for gain-phase, does have a small parasitic capacitance. This actually severely limits my frequency range significantly.

[Andrew_K]

You have a result of:
[A∗1+B∗−Y A∗0+B∗1]
[C∗1+D∗−Y C∗0+D∗1]

after multiplying the ABCD matrix:

[A B]
[C D]

Times the matrix for a shunt Y, which is:

[1 0]
[Y 1]

How did the result end up with the minus sign in front of the Y?  I don't get that; I get:

[A+BY   B]
[C+DY   D]

My measured ABCD was ABCD of the DUT, as well as the shunt.

I multiplied by the inverse matrix of the shunt to remove the contribution of the shunt.

Inverse matrix of the shunt is \begin{bmatrix} 1 & 0\\ -Y & 1 \end{bmatrix}

[The Electrician]

Why are you making your impedance measurements with a VNA rather than the 4990A?

[Andrew_K]

I don't have direct access to the 4990A, not for a while anyway.  Also, it is an autobalancing bridge device. As such, it cannot take balanced measurements. The ports are unbalanced, as the LCur is a virtual ground driven by an amplifier.

The VNA has impedance analysis functionality, and as I understand, should give better result for balanced measurements. It's also accurate enough for the impedance range and frequency range I'm interested in.

I tried using baluns, but the results I was getting were garbage. Even with significant effort in calibration.

[The Electrician]

I don't have direct access to the 4990A, not for a while anyway.  Also, it is an autobalancing bridge device. As such, it cannot take balanced measurements. The ports are unbalanced, as the LCur is a virtual ground driven by an amplifier.

The VNA has impedance analysis functionality, and as I understand, should give better result for balanced measurements. It's also accurate enough for the impedance range and frequency range I'm interested in.

I tried using baluns, but the results I was getting were garbage. Even with significant effort in calibration.

Given the fact that a VNA doesn't do well when circuit impedances are substantially different from 50 ohms, trying to get the 4 measurements for deriving an ABCD matrix using a VNA might be expected not to give good results.

With an open on one port, the impedance at the other might be expected to be about as high as possible.  Similarly, when there's a short on one port, the impedance at the other might be as low as possible.

Not a happy situation.

Just to satisfy my curiosity, what are the 4 measurement results you got on DUT1, at what frequency?

[Andrew_K]

I took some measurements using all methods discussed.
1) Gain Phase and Impedance
2) 1-Port Impedances using the impedance analysis functionality (S ports and GP ports offer this function)
3) S-Parameter to ABCD using math functions

S-Parameter had some obvious inaccuracies due to mismatch. Gain-Phase gave poor results, as the probe capacitance was too high (~0.5pF active differential probe).

1-Port impedance gave the best results by far.

Given the fact that a VNA doesn't do well when circuit impedances are substantially different from 50 ohms, trying to get the 4 measurements for deriving an ABCD matrix using a VNA might be expected not to give good results.

That is true if I use the S ports for 2-port analysis. There is obvious mismatch uncertainty in my results.

I don't know if the VNA is using math to decouple things, or if there is something hardware based on the S ports, but it is intended for impedance analysis. It's one of the features of this analyzer.

In the impedance measurements I took today, I didn't use the shunt. I took impedance measurements with both the S-ports, and the gain-phase ports (both are supported as impedance ports). The measurements agreed fairly well. I'm noticing that the Gain-Phase had better impedance measurement stability at high frequency, while the S ports had better impedance measurement stability at low frequency.

So far, the ABCD parameters look much better than when I tried using a shunt to preserve the open impedance at low frequencies.
The fact that the ABCD parameters agree, using two different methods of impedance measurement, give me confidence in the measurements.


(DUT1 B parameter for reference, both curves were obtained using different impedance measurement techniques)


Also, now the calculated cascade [DUT1] * [DUT2] is approximately equal to the measurement of [DUT1 & DUT2]


(B parameters of [DUT1&2] and [DUT1]*[DUT2] for reference)

I think with some clever tricks, like forcing reciprocality (and symmetry where applicable), curve fitting, and some averaging, these 2-port parameters will do what I want.

[The Electrician]

Again, to satisfy my curiosity, what is the manufacturer and model of the VNA?

[Andrew_K]

Keysight E5061B

[The Electrician]

Keysight E5061B

I want one of those.  Are you a student, and that gives you access to the E5061B at school, or is your project something you're doing as part of your job?

I went looking for my derivation of those  ABCD formulas, and I found the file that contains the ones I gave you, and some others.

In particular I found that I had derived a set that required measurement of a transfer function, but I never tried it out because I don't have a gain/phase analyzer (and I'm retired, so I no longer have access to one at work).

I derived these formulas using Mathematica to solve for them.  The next image shows the derivation, which is a fairly easy one.  There is a variable name "fvtfr" that stands for "forward voltage transfer ratio".  As you can see, the expressions are simple and they don't involve any measurements of a port impedance with the other port open circuited; they only involve the short circuited impedances.  These formulas might work very well for you.  Let me know if you try them and what results you get.  Here are the formulas:

[Andrew_K]

It's technically a work project. But learning about transfer functions, 2-port analysis, has been a personal learning goal of mine for a while. Just so happened that some of the things I've been learning about apply nicely to work.

I may have found a better way to calculate the transfer functions given these impedance measurements.

By reciprocity, $$Z12 = Z21$$ and $$Y12 = Y21$$

Using

$$Z_{IS} = \frac{1}{Y_{11}}, Z_{OS} = \frac{1}{Y_{22}}, Z_{IO} = Z_{11}, Z_{OO} = Z_{22}$$

$$ \begin{bmatrix} Y_{11} & Y_{12} \\ Y_{21} & Y_{22} \end{bmatrix} = \frac{1}{det(Z)} * \begin{bmatrix} Z_{22} & -Z_{12} \\ -Z_{21} & Z_{11} \end{bmatrix} $$

then for Z12 and/or Z21,

$$ Z_{11} * Z_{22} - Z_{12} * Z_{21} = \frac{Z_{22}}{Y_{11}}$$

$$ Z_{11} * Z_{22} - \frac{Z_{22}}{Y_{11}} = Z_{12} * Z_{21}$$

Since $$Z_{12} = Z_{21}$$,

$$Z_{12} = Z_{21} = \pm \sqrt{Z_{11} * Z_{22} - \frac{Z_{22}}{Y_{11}}}$$


I feel like this should be similar (or the same) mathematically. Yet, the results are much better numerically. There are far fewer random discontinuities.

Only "issue" is that the angle may be offset after the square root operation. This is corrected fairly easily by complex multiplication.

Then, converting to ABCD or H parameters, gives a result that simulates very well in QUCS.

[The Electrician]

You have: zis=z11, zos=z22, zio=y11, zoo=y22
but this is wrong.  It should be:

zis=1/y11, zos=1/y22, zio=z11, zoo=z22

When I want to verify this sort of thing, I draw a schematic of some simple resistor network and calculate the Y and Z parameters, then see if the equalities like those above are true.

For example consider a simple T network consisting of, left to right, a 3 ohm series resistor, a 5 ohm shunt resistor, and a 7 ohm series resistor.  The Z parameters for that network are:

[8 5]
[5 12]

and the Y parameters are the inverse of the Z matrix:

[12/71 -5/71]
[-5/71 8/71]

Clearly, zio is equal to 8, which is z11.
zoo is equal to 12, which is z22.

zis is equal to 3 + 5||7, which is 71/12, and that is equal to 1/y11.
zos is equal to 3||5 + 7, which is 71/8, and that is equal to 1/y22.

[The Electrician]

It's technically a work project. But learning about transfer functions, 2-port analysis, has been a personal learning goal of mine for a while. Just so happened that some of the things I've been learning about apply nicely to work.

If you're serious about this, you should buy this book: https://www.amazon.com/Electronic-Engineering-Applications-Two-Port-Networks/dp/148317154X/ref=sr_1_1?crid=9ZDR89KUB7EM&keywords=electronic+engineering+applications+of+two+port+networks&qid=1659629785&s=books&sprefix=electronic+engineering+applications+of+two+port+networks%2Cstripbooks%2C118&sr=1-1

And this one: https://www.amazon.com/Circuits-Matrices-Electrical-Engineering-Paperback/dp/B011MDTJUU/ref=sr_1_2?crid=258BXP6FK4H9E&keywords=circuits+matrices+and+linear+vector+spaces&qid=1659630033&s=books&sprefix=circuits+matrices+and+linear+vector+spaces%2Cstripbooks%2C114&sr=1-2

[Andrew_K]

You have: zis=z11, zos=z22, zio=y11, zoo=y22

  Typed it out wrong as I was copying from my notes and trying to format in LaTeX for the post.

It's technically a work project. But learning about transfer functions, 2-port analysis, has been a personal learning goal of mine for a while. Just so happened that some of the things I've been learning about apply nicely to work.

If you're serious about this, you should buy this book: https://www.amazon.com/Electronic-Engineering-Applications-Two-Port-Networks/dp/148317154X/ref=sr_1_1?crid=9ZDR89KUB7EM&keywords=electronic+engineering+applications+of+two+port+networks&qid=1659629785&s=books&sprefix=electronic+engineering+applications+of+two+port+networks%2Cstripbooks%2C118&sr=1-1

And this one: https://www.amazon.com/Circuits-Matrices-Electrical-Engineering-Paperback/dp/B011MDTJUU/ref=sr_1_2?crid=258BXP6FK4H9E&keywords=circuits+matrices+and+linear+vector+spaces&qid=1659630033&s=books&sprefix=circuits+matrices+and+linear+vector+spaces%2Cstripbooks%2C114&sr=1-2

Thanks for the recommendations! Definitely going to check these out.

[The Electrician]

Regarding the formulas I posted in reply #25, I have mentioned that since only 3 impedance measurements are needed to get the 4 ABCD parameters, one of the impedance measurements can be unused.  In those formulas, it was zio that went unused.

Here is another set of formulas that does use zio, and neglects zoo:



If these equivalencies: zis=1/y11, zos=1/y22, zio=z11, zoo=z22
are used in the final formula you derived for z12 in reply #44, and after some algebra, you will arrive at the result for C in the set of formulas just above because C = 1/z12

Try the set of formulas in this post.  You should get the same results you get with your derivation for z12 in reply #44, which have fewer discontinuities.

[The Electrician]

Andrew_K, what's happening?  Are you getting any good results?

in one of your posts you show how you have hooked and measured a couple of two-ports labeled "DUT1" and "DUT2".  Does one of those include that high resistance cable you described early on?  If so, does that mean that the other DUT only contains passive components capacitors, inductors and resistors connected in a network?  Can you post a schematic of what's in that particular DUT?