Does Kirchhoff's Law Hold? Disagreeing with a Master

[radioactive]

I tried to organize my thoughts about the whole thing here https://grumpyengineering.wordpress.com/ (only one post there for now) if you want to read them.

I'm looking forward to reading more of your thoughts if you continue.  Very easy to read.  I also echo your thanks to sredni and bsfeechannel, and especially Lewin for their responses/patience to the challenge put forth.  Combing those responses with working on an EM sim and being able to see the mag/dir of the fields over time/space definitely gave me a much better intuitive feel for it.

[ogden]

I tried to organize my thoughts about the whole thing here https://grumpyengineering.wordpress.com/ (only one post there for now) if you want to read them.

When you talk about advanced stuff like Faraday's law, you shall not ignore other laws like law of conservation of energy.

Pay close attention to following post made by proponent of Dr.Lewin to see where exactly you and Dr.Lewin made mistake.

[mhz]

I tried to organize my thoughts about the whole thing here https://grumpyengineering.wordpress.com/ (only one post there for now) if you want to read them.

When you talk about advanced stuff like Faraday's law, you shall not ignore other laws like law of conservation of energy.

I agree.

Pay close attention to following post made by proponent of Dr.Lewin to see where exactly you and Dr.Lewin made mistake.

I don't see an issue here, but feel free to point it out if you like.

[ogden]

Pay close attention to following post made by proponent of Dr.Lewin to see where exactly you and Dr.Lewin made mistake.

I don't see an issue here, but feel free to point it out if you like.

Your (and Dr.Lewin's) equation does not separate EMF (energy) source from load, incorrectly and blatantly saying that KVL is as follows:



Kirchsoffs CIRCUIT law requires circuit consisting of energy source and load. Correct equation would be:

[mhz]

Pay close attention to following post made by proponent of Dr.Lewin to see where exactly you and Dr.Lewin made mistake.

I don't see an issue here, but feel free to point it out if you like.

Your (and Dr.Lewin's) equation does not separate EMF (energy) source from load, incorrectly and blatantly saying that KVL is as follows:

It's not "my" equation. It's how Lewin defines KVL. I'm not sure why you think it can't be rewritten to explicitly show sources and loads. It would look like this.

Kirchsoffs CIRCUIT law requires circuit consisting of energy source and load.

Not really. I can apply KVL just fine to a trivial network of resistors with no energy source and still get the correct answers.

Correct equation would be:

Actually, I think there's a minus sign missing on the right side of the above equation, due to Lenz law, but I'm not really sure what you're arguing here. Are you saying that Lewin's definition of KVL doesn't work in this circuit? If so then yes, I agree, that's just about his entire point!

[ogden]

It's not "my" equation. It's how Lewin defines KVL. I'm not sure why you think it can't be rewritten to explicitly show sources and loads. It would look like this.

Right. BTW where I did say that I think it can't be rewritten? Look, whole idea of "KVL does not work" proof is based on statement that integral of E.dl for the loop equals zero, thus EMF equals zero which is as Dr.Lewin say impossible. Indeed it is impossible - because equation is incomplete, thus statement is futile. You just corrected it by writing EMF + ( -I*R ) = 0. If you agree then we are done. You disproved Dr.Lewin.

[mhz]

It's not "my" equation. It's how Lewin defines KVL. I'm not sure why you think it can't be rewritten to explicitly show sources and loads. It would look like this.

Right. BTW where I did say that I think it can't be rewritten?

When you said "Your (and Dr.Lewin's) equation does not separate EMF (energy) source from load". I suppose you didn't explicitly say it can't be rewritten, but you seemed to be implying that for some reason it couldn't. Anyways, I'm glad we agree on this.

Look, whole idea of "KVL does not work" proof is based on statement that integral of E.dl for the loop equals zero, thus EMF equals zero which is as Dr.Lewin say impossible.

In a typical batteries+resistors type circuit, the EMF is the batteries, and they are included in the E•dl integration. I wrote this out explicitly in my last post where the source and loads were specified in separate integrations (i'm not even sure if this is proper notation but I think you get the point). Saying that E•dl equals zero means the EMF must equal zero is not correct, and I don't believe Lewin ever said that (please link with timestamp if he did). Rather, the point is that using KVL (as defined by Lewin as E•dl = 0) will yield the wrong answer in the presence of time varying magnetic flux, and the reason it yields the wrong answer is that now we have an EMF that doesn't come from an electric field.

Indeed it is impossible - because equation is incomplete, thus statement is futile.

Of course it's incomplete, that's Lewin's point. And the way to complete it is to update it to Faraday's law. If you disagree I'd ask you how you would complete it.

You just corrected it by writing EMF + ( -I*R ) = 0. If you agree then we are done. You disproved Dr.Lewin.

As I mentioned in the previous post, there was a minus sign missing. In the drawing, the assumption is we're looking at a specific point in time where the emf from the solenoid is  1V. The value of the evenly distributed resistance will determine the value of the current (if it's total 1ohm, we get 1A, etc.). Either way, if you start from Faraday's law, you have 1V on both sides of the equation (both sides better be the same value otherwise we either screwed up or Faraday's law is somehow wrong). If you move that 1V over to the left then you're effectively saying 1V - 1V = 0V. Not a very enlightening statement and the spirit behind Lewin's "5 + 3 - 8 = 0" video.

I'm wondering if maybe this will help. Here I've rearranged Faraday's law for the bseechannel example to have the emf on left side (the source), and the load on the right side. Note both sides are still equal to E•dl and non-zero, and as always you can subtract the RHS side from both sides if you want to see zero there.


In addition to asking how you would complete Lewin's KVL (Int E•dl = 0) to make it correct (I agree that it is not universally correct), I think I should ask you to clarify exactly what it is that you think Lewin has done incorrectly so we can make some progress in understanding each other.

[ogden]

Of course it's incomplete, that's Lewin's point. And the way to complete it is to update it to Faraday's law. If you disagree I'd ask you how you would complete it.

That's the whole point - you cannot use incomplete equation to prove anything! Integral E.dl = 0 of Kirchoff's circuit rule includes *both* EMF source and load. Integral E.dl of Maxwell's equation includes/describes only EMF *source*. You completed it for me:

In addition to asking how you would complete Lewin's KVL (Int E•dl = 0) to make it correct (I agree that it is not universally correct), I think I should ask you to clarify exactly what it is that you think Lewin has done incorrectly so we can make some progress in understanding each other.

Move right side (load) of equation you just completed to left side and I am done showing where Dr.Lewin was wrong. It will be in front of your eyes contradicting with what you say in your blog:

[mhz]

Of course it's incomplete, that's Lewin's point. And the way to complete it is to update it to Faraday's law. If you disagree I'd ask you how you would complete it.

That's the whole point - you cannot use incomplete equation to prove anything! Integral E.dl = 0 of Kirchoff's circuit rule includes *both* EMF source and load. Integral E.dl of Maxwell's equation includes/describes only EMF *source*. You completed it for me:

In addition to asking how you would complete Lewin's KVL (Int E•dl = 0) to make it correct (I agree that it is not universally correct), I think I should ask you to clarify exactly what it is that you think Lewin has done incorrectly so we can make some progress in understanding each other.

Move right side (load) of equation you just completed to left side and I am done showing where Dr.Lewin was wrong. It will be in front of your eyes contradicting with what you say in your blog:

If I move the right side of the equation to the left hand side, it will look like this:



So what?

It doesn't contradict anything I wrote in that blog. The point there is that something can't be simultaneously zero and non zero. This is how Lewin points out that EMFs don't have to come from batteries and then introduces Faraday's law. Maybe it will help if you look at this to get the full picture:



I feel like perhaps your disconnect is that you feel that the EMF in the Lewin circuit must be able to be part of the int E•dl term, but it never will be because it comes from a time varying magnetic flux. int E•dl is *not* (necessarily) the same thing as the sum of all EMFs and voltage drops in the loop! It is however *always* equal to the negative time rate of change of the magnetic flux, which in this demo is the *only* emf, i.e. the only thing causing anything to happen (this is Faraday's law, and I am just assuming all this time that you agree with Faraday's law, please let me know if this is not the case). Think of the changing magnetic flux as the source, and int E•dl as the way you can figure out what is happening in the load. In this circuit we've been talking about int E•dl is nothing but currents through resistors. You can add a real battery in there and then you'll have two different kinds of EMF but that's not what we've been talking about up til now.

I'll ask once more for you to clearly articulate what it is that you think that Lewin got wrong. Please don't just respond to something I said here, state your objection clearly so that I can respond to it (tomorrow, we're in very different time zones). I'm still not totally clear on what your objection is but it feels like we might be able to come to an understanding.

[ogden]

The point there is that something can't be simultaneously zero and non zero.

It can - when "something" is one thing in one case and completely different in another.

Take following as my objection you asked for: you cannot take in account law of conservation of energy in KVL case but ignore it in Maxwell's.

Shall I repeat & emphasize : Integral E.dl part of Kirchoff's circuit rule includes *both* EMF source and load. Integral E.dl of Maxwell's equation includes/describes only EMF *source*. You simply can't equal two (integrals), because they "look the same" (your words BTW). Following equation describes circuit of Dr.Lewin's experiment inner loop:



Don't you find it similar to equation of KVL you wrote?



I assume you agree to both. Me too.

[mhz]

OK now we're getting somewhere, i think (i hope!). If I understand you correctly, you believe that Faraday's law implies a violation of conservation of energy!

"you cannot take in account law of conservation of energy in KVL case but ignore it in Maxwell's." I would call this a strawman, unless you can point me to where Lewin said that Maxwell's equations require ignoring conservation of energy.

It's his KVL that violates conservation of energy in his experiment because it can't possibly account for the source of energy as it knows nothing about magnetic flux!

I'm not sure I'm going to be able to bring you over without repeating things I've already said in the past few posts, but I'll try.

The point there is that something can't be simultaneously zero and non zero.

It can - when "something" is one thing in one case and completely different in another.

but.. it's not. int E•dl is int E•dl is int Ed•dl. It has the same meaning in both Lewin's KVL and Faraday's law. It means you go around a loop adding up each incremental bit of E field you encounter along your path. It doesn't matter whether it's an E field from a battery or an E field in a resistor or an E field arising from a changing magnetic flux. Do you believe that int E•dl has somehow a different meaning in the two equations?

Take following as my objection you asked for: you cannot take in account law of conservation of energy in KVL case but ignore it in Maxwell's.

Shall I repeat & emphasize : Integral E.dl part of Kirchoff's circuit rule includes *both* EMF source and load. Integral E.dl of Maxwell's equation includes/describes only EMF *source*.

I would say that as applied to this experiment, Faraday's law equates Int E•dl with the source (negative time rate of change of magnetic flux through the loop) of energy. It seems like you are unwilling to accept an EMF that doesn't arise from a source that is clearly related to an E field being maintained between two points, and that's what's causing you trouble, but that's the reality that Faraday describes.

You simply can't equal two (integrals), because they "look the same" (your words BTW).

I think you are misunderstanding my point. Let's use completely different symbols to hopefully make it clear. Let's look at two equations:

Y = 0

Y = F(x)

In equation one, we are saying that Y = 0. In equation two we are saying Y is equal to some function of x. There is only one way both can be valid   and that is the case where F(x) = 0 for any value of x. But we are asserting that F(x) also takes on non-zero values. Therefore Y = 0 is not a universal relation but just one possible point on the real general case of Y = F(x). 0 is just one possible value in the range of the function.

If it helps, think of Y as your height above the ground, and F(x) as a function that gives your current height above the ground. You might spend days, weeks or months walking around thinking that F(x) always equals 0 because you're always firmly on the ground. Then you get in an elevator, or an airplane for the first time. F(x) is no longer zero, and you must conclude that Y = 0 is a special case and not a general relation. This is mathematically, logically the same argument with different symbols.

Following equation describes circuit of Dr.Lewin's experiment inner loop:



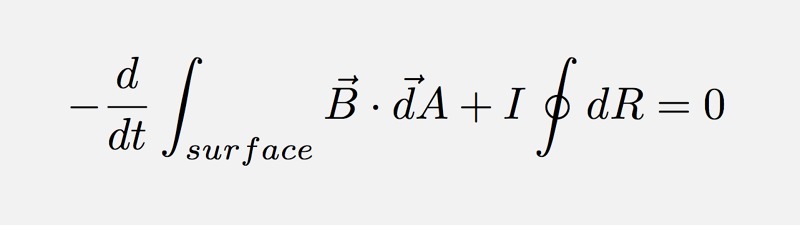
Don't you find it similar to equation of KVL you wrote?



I assume you agree to both. Me too.

Yes, and I had to use Faraday's law to get there. Lewin's KVL could not have done it. That is one of his points.

[Edit: few clarifying words]
[Edit2: fix broken quoting]

[ogden]

OK now we're getting somewhere, i think (i hope!). If I understand you correctly, you believe that Faraday's law implies a violation of conservation of energy!

"you cannot take in account law of conservation of energy in KVL case but ignore it in Maxwell's." I would call this a strawman, unless you can point me to where Lewin said that Maxwell's equations require ignoring conservation of energy.

It's his KVL that violates conservation of energy in his experiment because it can't possibly account for the source of energy as it knows nothing about magnetic flux!

Seems, you did not get it or just pretend that you do not understand what I did mean with that sentence.

Ok. Next try. I do not talk about abstract KVL and Maxwell equation "cases". I talk about equations that describes circuit of experiment. Everything seemingly is ok with KVL simple int E.dl = 0, yet I would prefer to split it into EMF source and load, as you already did - thank you for that. Problem arises when Dr.Lewin use plain Maxwell's equation and say that it miraculously tells everything about inner loop of his experiment. I disagree. Maxwell's equation is just EMF source part! Where's physics of load (resistors) in Maxwells equation? If you leave it like that, then it is indeed violation of conservation of energy. Plain Maxwell's equation can be used only to describe superconductive ring (w/o embedded resistors) placed in changing magnetic flux.

I would say that as applied to this experiment, Faraday's law equates Int E•dl with the source (negative time rate of change of magnetic flux through the loop) of energy. It seems like you are unwilling to accept an EMF that doesn't arise from a source that is clearly related to an E field being maintained between two points, and that's what's causing you trouble, but that's the reality that Faraday describes.

Oh my. You better take care of your own troubles first, ok? Seems, you are unwilling to accept that EMF energy is dissipated in the embedded resistors of the inner loop.

[mhz]

If you don't believe Maxwell's equations always apply, or you think they violate conservation of energy, then I'm afraid we can't go anywhere from there. Maxwell's equations are axiomatic to any discussion about electricity and magnetism regardless of circuit configuration (regardless of whether the elements of the circuit can be  represented as lumped elements or not) and they always hold[1].

Still, thank you for the conversation. It's been helpful to me.

[edit: added bit in the parenthesis]
[edit2: minor clarifying edit]
[edit3: add bit about QED]

[1] Except, apparently in the domain of Quantum Electrodynamics, which is not what we're talking about here.

[ogden]

If you don't believe Maxwell's equations always apply, or you think they violate conservation of energy, then I'm afraid we can't go anywhere from there.

LOL. Here we go. Again. When out of arguments - just state that debate opponent does not understand Maxwell's equations. 

I never said or implied that I do not believe Maxwell's equations. Also I never said that they violate law of conservation energy. What I did say that you can't use EMF source equation alone to describe system of EMF source and load. If you cannot comprehend such a simple concept then further discussion indeed is pointless. Funny that you even did write proper equation which shall be used to describe/analyze circuit of experiment against "KVL apply to circuit or not", but when you most likely realized that it would destroy your whole system of beliefs/reasoning, you decided to completely ignore everything about it:

[mhz]

LOL. Here we go. Again. When out of arguments - just state that debate opponent does not understand Maxwell's equations. 

I never said or implied that I do not believe Maxwell's equations. Also I never said that they violate law of conservation energy.

You said:
"Maxwell's equation is just EMF source part! Where's physics of load (resistors) in Maxwells equation? If you leave it like that, then it is indeed violation of conservation of energy. Plain Maxwell's equation can be used only to describe superconductive ring (w/o embedded resistors) placed in changing magnetic flux."

I can't go anywhere from there. If you think that you can write something like "Plain Maxwell's equation can be used only to describe ..." then either you think that there are more fundamental equations or you simply don't understand how they work. Maxwell's equations are the starting point. Everything else can be derived and approximated from them. This is accepted science for the last 100+ years.

It's clear that you disagree with me and that's fine. I'm willing to end this discussion in disagreement. I don't think there's anything left for me to say, but as I said I appreciate the discussion.

p.s. the load (resistors) are in the int E•dl. it's all up there in the equations i've already posted.
[edit: p.p.s. i'd been waiting for the emoticons to come out. not a device i'd employ if I wanted to be taken seriously]

[ogden]

I can't go anywhere from there.

Yes. Please. Stop this nonsense of pretending that you do not understand what I mean. Our discussion looks like broken record.

[bsfeechannel]

LOL. Here we go. Again. When out of arguments - just state that debate opponent does not understand Maxwell's equations. 

You don't. But that's not your fault. Maxwell's equations show how Nature is much weirder than we may conceive. You'll have to reboot your brain to understand it. Just give it time. We all had our Maxwell crisis.

You're just having yours in public.

[mhz]

LOL. Here we go. Again. When out of arguments - just state that debate opponent does not understand Maxwell's equations. 

You don't. But that's not your fault. Maxwell's equations show how Nature is much weirder than we may conceive. You'll have to reboot your brain to understand it...

Or in my case literally spend a few months of sleepless nights reading/watching everything on the topic. There's still a gremlin in my understanding but I'll start a different thread for that one.

I can't help but feel all of the drama around this could have been avoided if electronics educators did a better job of adding caveats and asterisks to their materials and explanations. I went to UC Berkeley and I'm pretty sure not a single professor ever uttered the words "lumped circuit abstraction" in my entire undergrad career. Agarwal is doing God's (or rather Feynman's) work.

[electrodacus]

I can't go anywhere from there.

Yes. Please. Stop this nonsense of pretending that you do not understand what I mean. Our discussion looks like broken record.

Do not waste your time.

[bsfeechannel]

I can't help but feel all of the drama around this could have been avoided if electronics educators did a better job of adding caveats and asterisks to their materials and explanations. I went to UC Berkeley and I'm pretty sure not a single professor ever uttered the words "lumped circuit abstraction" in my entire undergrad career. Agarwal is doing God's (or rather Feynman's) work.

Exactly.

Kirchhoff is normally taught in high school. Maxwell is mentioned only en passant. No one says that Maxwell is the theory underlying Kirchhoff's laws. When you get to college, you are taught a lot of apparently meaningless and complicated math. No one says that this will be in preparation for Maxwell and other theories. Then they teach you electromagnetism mercilessly without explaining that the fluxing Kirchhoff is a special case of Maxwell.

Lewin is a critic of that way of teaching, given how that cripples understanding the basic concepts.

We could suggest a change. Teach a simplified version of calculus and vector analysis in high school. This is perfectly possible. My video "Calculus for young players" is aimed at high-schoolers. Then teach the basics of Maxwell, explaining that Kirchhoff is a special case of that theory.

When at college, they should say, now that you are going to be an engineer, you will be responsible for designing serious things. So you are going to learn this stuff with all the rigor these theories require. You'll also learn circuit analysis with even more detail and rigor, always having in mind that it is a special case of electromagnetism.

That would avoid the anger everybody feels when they discover the truth years later.

[Berni]

This is proabobly the most important realization you made Mhz:
https://grumpyengineering.wordpress.com/

If you still disagree with the above I would love to hear from you, but I believe, most of the confusion results from disagreements about what KVL is.

Nobody in this thread is trying to prove that Maxwells equations are wrong, stop blaming people for that.

Most of the arguing between the "Kirchoff" and "Maxwell" sides is due to both sides having a different idea of what KVL is. I fully agree that if KVL is what Dr. Lewin explains it as being then its garbage as soon as you have changing magnetic fields. I have no idea where he got that definition of KVL, everywhere i see it defined as the flowing:

The algebraic sum of all the voltages around any closed loop in a circuit is equal to zero

It explicitly mentions all voltages, this includes the electric field as well as the EMF (I think we all agree EMF is a voltage). Also notice that it mentions an algebraic sum (This is not an integral!) since KVL is not a law of the universe but a tool for analyzing circuit mesh models.

Both the "Kirchoff" and "Maxwell" sides are correct! The only only reason that we are arguing is because the so called "Maxwell" side is using a less useful interpretation of KVL. The people on the "Kirchoff" side are not denying anything about Maxwells equations, the only thing this side is trying to say is that you can use KVL to solve Lewins paradoxic circuit just fine i you use KVL correctly. You indeed CAN NOT USE Kirhoffs laws for everything, but this particular circuit is not such a case.
 
The "Kirchoff" side should actually be named "Kirchoff and Maxwell" side. We are happy to use one or the other rather than swear by Maxwell only, just a matter of the right tool for the job. Is there something wrong with that?

[bsfeechannel]

Nobody in this thread is trying to prove that Maxwells equations are wrong

But that is exactly what you do when you say that you can solve Lewin's circuit with Kirchhoff. Which, by the way, is not Lewin's. You find that circuit anywhere because it defines the phenomenon of induction.

Your claims lead immediately to a logical contradiction which indicates that your reasoning is false.  And that's why people keep "blaming" you, or better, warning you.

[Berni]

Circuit analysis makes use of equations derivated from Maxwells equations in order to make inductors and capacitors work with Kirchoffs circuit laws. Is there some law that forbids the use of Maxwells equations in anything else but there raw form? Somehow making anything they are used in automatically wrong even if it gives the same result?

So is it a problem that Kirhhoffs cirucit laws work in lumped circuit meshes? Or is it a problem that circuit modeling has to be used to link the real world with circuit meshes?

[mhz]

This is proabobly the most important realization you made Mhz:
https://grumpyengineering.wordpress.com/
Quote

    If you still disagree with the above I would love to hear from you, but I believe, most of the confusion results from disagreements about what KVL is.


Nobody in this thread is trying to prove that Maxwells equations are wrong, stop blaming people for that.

On the contrary, that's exactly where the previous discussion with ogden led, with them concluding that Maxwell's equations are only correct in specific situations (superconducting rings, I believe is what was said). That's another way of saying that sometimes they are wrong.

Most of the arguing between the "Kirchoff" and "Maxwell" sides is due to both sides having a different idea of what KVL is. I fully agree that if KVL is what Dr. Lewin explains it as being then its garbage as soon as you have changing magnetic fields. I have no idea where he got that definition of KVL, everywhere i see it defined as the flowing:
Quote

    The algebraic sum of all the voltages around any closed loop in a circuit is equal to zero

the problem with this definition, what I keep calling the "modified" KVL, which is what I think most of us think of as the "real" KVL,  is it starts to break down in situations where you're dealing with nonlumped elements. As I mentioned in the blog post, there is nothing keeping us from modeling things with lumped elements (we'd have to add a mutual conductance in the inner loop) to get thngs to sum to zero in a nice KVL way but at the expense of incorrectly localizing the effect.

It explicitly mentions all voltages, this includes the electric field as well as the EMF (I think we all agree EMF is a voltage). Also notice that it mentions an algebraic sum (This is not an integral!) since KVL is not a law of the universe but a tool for analyzing circuit mesh models.

right! the algebraic sum is the key to pointing out that it only works with lumped circuits, or circuits modeled with lumped elements.

Both the "Kirchoff" and "Maxwell" sides are correct! The only only reason that we are arguing is because the so called "Maxwell" side is using a less useful interpretation of KVL. The people on the "Kirchoff" side are not denying anything about Maxwells equations, the only thing this side is trying to say is that you can use KVL to solve Lewins paradoxic circuit just fine i you use KVL correctly. You indeed CAN NOT USE Kirhoffs laws for everything, but this particular circuit is not such a case.

I'd invite you to redraw the diagram and use the "modified" KVL on this circuit. See if you can model it in a way that doesn't falsely localize the effect of the mutual inductance but that allows you to get the correct answer with the "modified" KVL. It would help if you post the drawing of the updated model for us.

The "Kirchoff" side should actually be named "Kirchoff and Maxwell" side. We are happy to use one or the other rather than swear by Maxwell only, just a matter of the right tool for the job. Is there something wrong with that?

Nothing wrong with that at all. The real lesson of all this, to me at least, is that the "modified" KVL only works with lumped circuits. If Lewin had introduced the concept of lumped circuit analysis (as described expertly by Feynman and Agarwal) explicitly after the super demo I think there would be probably be a lot less confusion.

[Berni]

I'd invite you to redraw the diagram and use the "modified" KVL on this circuit. See if you can model it in a way that doesn't falsely localize the effect of the mutual inductance but that allows you to get the correct answer with the "modified" KVL. It would help if you post the drawing of the updated model for us.

I already did that in the very first few posts on this thread:
https://www.eevblog.com/forum/chat/does-kirchhoffs-law-hold-disagreeing-with-a-master/msg1945312/#msg1945312

You simply calculate the contributions each wire segment is providing and distribute the inductance accordingly (Note these are all coupled inductors since the all interact with the same flux). This way only parts of the circuit that are not interesting get lumped up while preserving all the points of interest along with there voltages. If you suddenly want to know whats happening half way along a wire you simply represent it as two lumps with correct proportions to expose the point we want.

Calculating the inductance is easy in this case because the circuit is very symmetrical and there is high coupling between the wires due to them flowing the same path. Had the wires followed arbitrary complex 3D paths trough space it would have been much harder to model. We would need to go trough Maxwell with fancy convoluted 3D space intergals in order to get the magical inductance values for the equivalent circuit. Or if we have access to the physical circuit simply measure them with a impedance analyzer and plonk the numbers into the equivalent circuit. No matter what the process is you end up with a cirucit mesh that acts like the real cirucit, but can be used with KVL just fine since its a lumped circuit mesh.

Modeling has nothing to do with KVL or even electronics. Its simply a process of turning a real thing into math that acts like the real thing so that we can run calculations or simulations on it. Its done with all sorts of things, be it mechanical, structural, thermal etc. These models all have there limitations. Just as a thermal model of a house will not be accurate if you try to simulate ambient being 10 000 °C in the same way your equivalent circuit will not be accurate at RF frequencies unless specifically modeled for it.